What I Learned Today

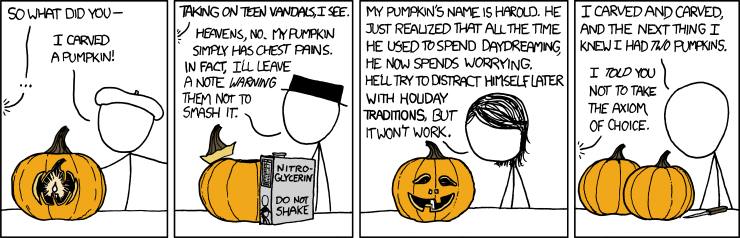
A recent xkcd comic featured a puzzling pumpkin-carving outcome: a single pumpkin turning into two!

Like 88% of Randall Munroe’s readers, I went straight to wikipedia to figure out what the “Banach-Tarski” reference in the title-text meant (the other 12% already knew). The Banach-Tarski paradox “… is a theorem in set theoretic geometry which states that a solid ball in 3-dimensional space can be split into a finite number of non-overlapping pieces, which can then be put back together in a different way to yield two identical copies of the original ball.”

This is a fun mental puzzle/paradox because it obviously violates our intuitive sense of geometry. The “trick” is that the pieces into which you carve the pumpkin are rather special. “They are not usual solids but infinite scatterings of points,” says wikipedia. I don’t think the knife that can make those cuts has yet been invented! These pieces are are “nonmeasurable sets”, for which there is no defined notion of volume, so it’s maybe unsurprising that volume might not be conserved. The relevant theorem, though, shows that you can rotate and reassemble these special pieces, without distorting any of them, and end up with two solid full-size copies of the original pumpkin.

A related Tarski-Banach theorem shows that you can similarly carve up any single object and reassemble it into any other object, so your pumpkin can turn into a car or a star or a bacterium (see this very readable summary and analysis).

The final part of the comic punchline arises from the fact that both Tarski-Banach results depend on assuming the Axiom of Choice. This axiom states that given a collection of sets X, it is possible to create a new set Y by picking one item from each of the sets in X. This seemingly straightforward axiom has had a controversial history, partly because it can lead to such unintuitive conclusions. Axioms, of course, are neither true nor false; they are the basic assumptions that one takes as given before proceeding to prove something else. If you include the Axiom of Choice in your base set, then you can proceed to proving the Tarski-Banach theorems. If not, then no matter how you carve your pumpkin, you’ll never accomplish any magic feats of duplication or transmogrification: instead, you’ll only have the same pumpkin you started with!